Cite as: “R.D. Pascual-Marqui: Discrete, 3D distributed, linear imaging methods of electric neuronal activity. Part 1: exact, zero
error localization. arXiv:0710.3341 [math-ph], 2007-October-17, http://arxiv.org/pdf/0710.3341 ”
Page 2 of 16
density; c
is a
scalar accounting for the physics nature of electric potentials
which are
determined up to an arbitrary constant; and 1 denotes a vector of ones, in this case
.
Typically
, and
.
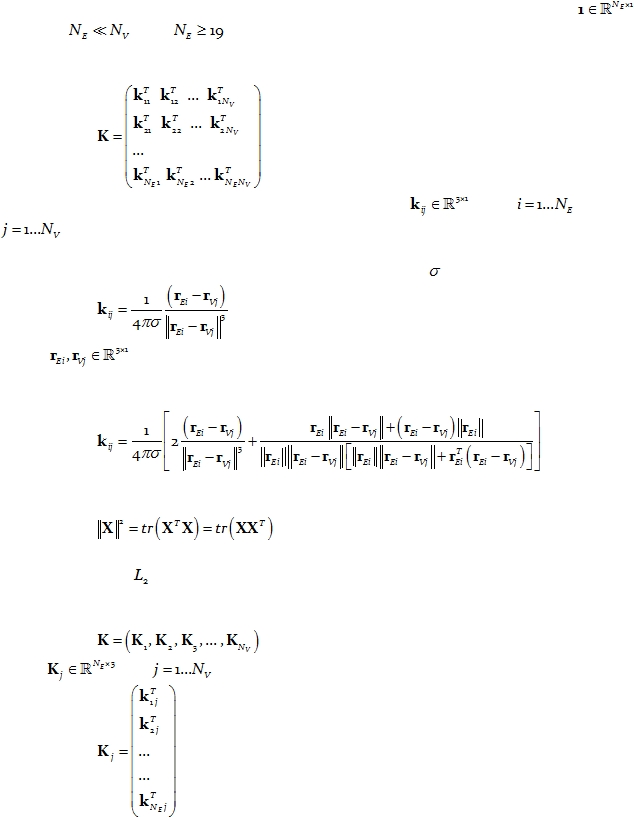
In Eq. 1, the structure of K is:
Eq. 2:
where the superscript “T” denotes transposition; and
, for
and for
, corresponds to the scalp potentials at the i-th electrode due to three orthogonal
unit strength dipoles at voxel j, each one oriented along the coordinate axes x, y, and z. For
instance, in infinite homogeneous medium with conductivity
:
Eq. 3:
where
are position vectors for the i-th scalp electrode and for the
j-th voxel,
respectively. As another example, for the case of a homogeneous conducting sphere in air,
the lead field is:
Eq. 4:
In the previous equations, the following notation was used:
Eq. 5:
where tr
denotes the trace, and X is any matrix or vector. If X
is a vector, then this is the
squared Euclidean
norm; if X is a matrix, then this is the squared Frobenius norm.
Note that K can also be conveniently written as:
Eq. 6:
where
, for
, is defined as:
Eq. 7:
Ideally, the lead field should correspond to the real head (with realistic geometry and
conductivities). For the EEG problem, the voxels should correspond to cortical grey matter.
For other situations (e.g. EKG), appropriate volume conductor models and solution spaces
should be used.